


During the Christmas puzzle week, which we bring you for the twentieth year in succession, you need to be prepared for puzzles that cannot be easily solved with a computer (the point of this endeavour). We give you tasks which require you to think all by yourself. But unlike in the past we will not be giving you a separate instalment every single day between Christmas and New Year. Just occasional entertainment during the holiday season.

Today we bring you a special puzzle by Werner Keym, who is one of the most creative problemists I know. He specializes in problems involving castling, en passant captures and pawn promotion. For many years I have enjoyed his problems, which I often encountered. Many have the advantage of not being prone to instant solution by chess engines. They force you to think. His most recent English language book, "Chess Problems out of the box," is going to keep me busy for a long time to come. It is one of the most entertaining works I have ever encountered (and it is competing with Loyd, Dawson, Fabel, Smullyan and co.). You can get yourself a copy here (€12/US $12 + postage €2/€4).

And now without further ado here is a Christmas puzzle Werner Keym sent me:
It looks impossible: Black can always escape White's attacks, at least for three moves, e.g. 1.♗xf5+ ♚xc7 2.♖g7+ ♝f7+ 3.♖xf7+ ♚b8 6.♖b7+; or 1.♖g7 ♚xc8 2.♗xf5+ ♝d7 3.♔d6 ♝xf5 4.♖g8#.
The only way to mate in three seems to be after capturing en passant on f6. But in chess problems that is only allowed if you can conclusively prove that Black's last move can only have been a double-step of the pawn: f7-f5. You must show that it was the only possible move, i.e. that any other Black move previous to the position above was not possible.
Let us make it absolutely clear: you need to find a position in which Black and White played one legal move each, and then in the diagram position Black played f7-f5, allowing White to play 1.e5xf6 e.p. and deliver mate on his third move. Can you find the previous two moves?
If you are getting a feel for this kind of problem, here are a few more. The following two are real classics — you see them everywhere. They are also fairly easy. The first is by Dr Niels Høeg from 1916, the second by Raymond Smullyan in 1980. In each case the question is what could have been the previous move or moves, how could these positions have occurred?
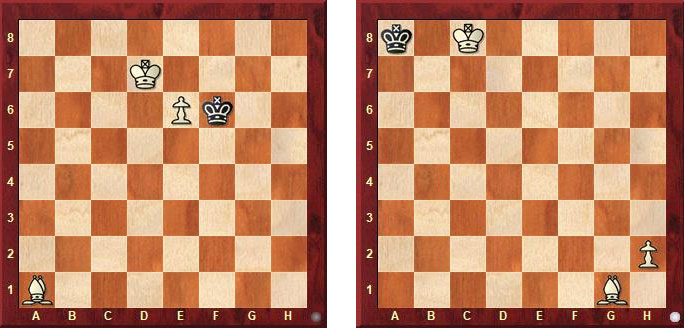
How did this happen — what were the last moves?
The next two are harder but very pleasing to solve:

How did this happen — what were the last moves?
In the first diagram (by Werner Keym, Die Schwalbe 1979) Black is in check. What were the previous moves? In the second diagram (by Niels Høeg, Skakbladet 1924) the question is how could that position have arisen? You must find a legal position that can lead to the above diagram in three moves.
The solution to these problems will be given in the third week of January.