Chess Logical: did you solve it?

by Frederic Friedel
5/21/2021 – It was a two-part task: from the reactions of students to a Zen master's whispered hints, they try to deduce which piece he is thinking of. In the second part you have to solve a chess study with a uniquely different drawing strategy. Very strong players all over the world joined in our experiment – most failed to solve the Logical. Here at last are the solutions – both of them.

ChessBase 16 - Mega package Edition 2021 ChessBase 16 - Mega package Edition 2021

Your key to fresh ideas, precise analyses and targeted training!
Everyone uses ChessBase, from the World Champion to the amateur next door. It is the program of choice for anyone who loves the game and wants to know more about it. Start your personal success story with ChessBase and enjoy the game even more.

More...

Christian Hesse explained the theory of such logical puzzles in his book "Achtung Denkfalle" (English: "Careful: thought trap"):

The Zen Chess Puzzle illustrates, in chess context, the idea that knowledge is not merely knowledge. Knowledge about a certain fact in a group of two people can mean that each person knows the fact. But it can also be the case that each person also knows that the other person knows the fact, and even that each person knows that the other person knows that the other person knows the fact. This is possible not only for knowledge, but also for the lack of knowledge and even for the impossibility of knowledge of a certain fact.

Our Zen Chess Logical illustrates that a transition can take place from the impossibility of knowing something (namely which piece the Zen Master is talking about) to the possibility of knowing this, paradoxically by someone merely stating the impossibility of this particular knowledge.

If you have difficulty with the solution to our Zen Logical, here's a little warm-up task that might help you understand the process:

Two logicians go to a cafeteria. The waitress comes to their table and says: "Coffee for both of you?" The first logician says "I don't know," and the second says "Yes." What does each want, and how did they figure it out?

The Zen Chess Logical

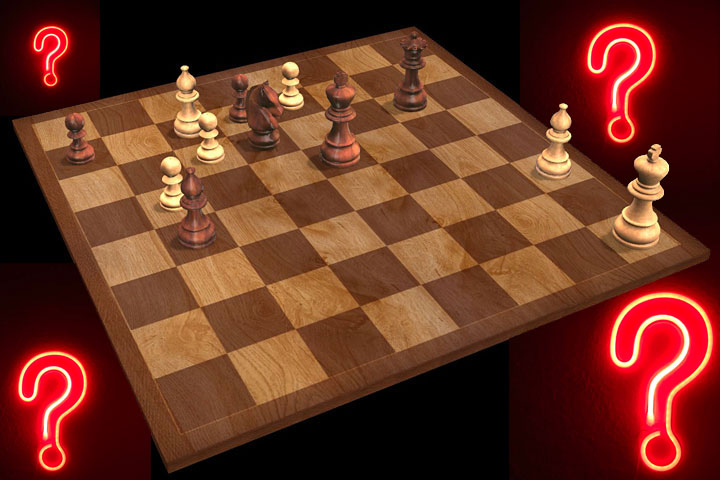
The logical problem we published two weeks ago was created by Christian Hesse, and is typical for his way of thinking. A Zen master visits a chess club and sees two students studying a chess position with the following material on the board:

The Zen master thinks of one piece and whispers some information into each student's ear. He tells Kaito which piece type he is thinking of, and Toshi the colour of the piece. Toshi is the first to react: "There is no way either of us could deduce which piece you mean." To which Kaito says: "Okay, now I know which piece it is!" Toshi: "Aha, then I know as well."

The chess logical was a task for our readers: find out which piece the Zen master meant, and how Kaito and Toshi could deduce it. So here's the solution:

  • Both Kaito and Toshi know that Kaito has been told the type and Toshi the colour of the piece.
  • Toshi immediately says nobody can know which one it is. 
  • From this Kaito deduces it must be white, because otherwise Toshi had to consider the possibility that it might be a knight or a queen, in which case Kaito would know which piece the Zen master was thinking of.
  • Since Kaito now says he knows which piece it is, Toshi deduces it must be the white king, because bishop or pawn would not specify the exact piece. So now Toshi also knows it was the white king.

A number of readers got it right, while we can tell you that so far many world-class players have been unable to find the answer to the Logical. With that they have helped us collect data for our attempt to answer the question: Are chess super-talents generally smarter than regular kids? So far only two young talents have given us the correct answer. And grown-ups? John Nunn solved it almost immediately, Ken Thompson too, followed by Vishy Anand. But not many more.

Professor Christian Hesse with one of the successful solvers

The Yakimchik study

The second task for our readers was to solve the study on the chessboard. It was given to us by Dr Karsten Müller (to match the Chess Logical material) and is cleverer than it looks at first sight. Here is the full solution, nicely annotated by our problem expert Siegfried Hornecker:

 

Have you seen this kind of drawing mechanism before? We hadn't.

<< Fighting for a spot in the Candidates: Gibraltar Women’s GP
The ChessBase News Review (07.05. - 21.05) >>
Editor-in-Chief emeritus of the ChessBase News page. Studied Philosophy and Linguistics at the University of Hamburg and Oxford, graduating with a thesis on speech act theory and moral language. He started a university career but switched to science journalism, producing documentaries for German TV. In 1986 he co-founded ChessBase.
Discussion and Feedback Join the public discussion or submit your feedback to the editors

See also

Hybrid Chess - an okay way to play during the pandemic

5/19/2021 – Playing over-the-board but entering the moves of the game into a computer to play online against an opponent who is playing in an entirely different location, all supervised by an arbiter - that is Hybrid Chess. After the first hybrid tournaments the players now share their impressions. But feedback from the Mitropacup participants reveals mixed attitudes about hybrid chess and the rules that were applied, writes Stefan Löffler. | Photo: Peter Sadilek | Photo: Stefan Löffler
More...
 5

See also

Mitropa Cup: Double success for Italy

5/14/2021 – Due to the pandemic, the Mitropa Cup 2021 was played as a "Hybrid Tournament" - the teams met at a central venue in their own countries from where they played online against the other teams, supervised by an arbiter. The Italian teams coped best with this still unusual form of tournament chess: they won gold in the Open Tournament and in the Women's Tournament. | Picture: Italian Chess Federation
More...
 2

Video

60m van Wely Anti Sicilian

Tired of spending hours and hours on the boring theory of your favourite opening? Then here is your solution, play an Anti-Sicilian with 3.Bb5 against 2...d6 or 2...Nc6, and 3.d3 against 2...e6. In 60 minutes you will get a crash course in how to avoid mainstream theory and in understanding the ideas of this Anti-Sicilian setup. After these 60 minutes you should be able to survive the Sicilian for a long time, without being bothered by new developments found by engine x supported by an x-core machine. Now that it finally comes down to understanding, let's play chess!

Discuss

Rules for reader comments

 
 

Not registered yet? Register

DanKwik DanKwik 5/22/2021 02:51
Toshi needs to know what Kaito knows first before he can make the deduction if not it would be impossible for him to know.
lagartodisecado lagartodisecado 5/21/2021 11:04
The White King (although not the ``correct'' choice for a Zen Master).

-The Master couldn't say black to Toshi, since otherwise Kaito would have known if he had been told that the piece was a knight.
-Once Toshi said that neither could guess, then Kaito knew it was a white piece. Since this information gave him the clue, it meant that there could be only one white piece of this type -> ie the king.
-After revealing that he had solved the problem, then Toshi could follow the exact same process to arrive to the conclusion.
JoshuaVGreen JoshuaVGreen 5/20/2021 03:46
I first saw that drawing mechanism in "Endgame Magic" by John Beasley & Timothy Whitworth:

V. Shoshorin
5th Honorable Mention, "Shakhmaty v SSSR," 1970
White to play and draw


Main line: 1. Bc5! c1Q 2. Nxc1 Bc3+ 3. Kf7 e1Q 4. Bd7+ Ka5 5. Nb3+ Ka6 6. Bc8+ Kb5 7. Bd7+ Kc4 8. Be6+ Kd3 9. Bf5+ Ke2 10. Bg4+ Kf1 11. Bh3+ etc.
Michael Jones Michael Jones 5/20/2021 01:24
Toshi knows that Kaito knows which kind of piece it is, and can deduce that that is not enough information for Kaito to know precisely which piece. If Toshi had been told that the piece was black, he could not be sure of this - if Kaito had been told that it was a queen or knight, he would immediately know which one since there is only one of each on the board. Therefore the piece must be white - and Kaito must have been told that it was one of a king, bishop or pawn. Kaito knows that Toshi must have followed that logic in making his first statement, so once Toshi makes it, Kaito knows that the piece must be white. If he had been told that it was a bishop or pawn, he would still not know which one since there are two white bishops and three white pawns - so his statement that he now knows which one implies that it must be the white king. Kaito's statement tells Toshi that he has followed that logic, so Toshi now also knows which one it is - although Toshi's second statement is not actually necessary for the solution; if he had said nothing further, the puzzler would still be able to deduce that it must be the white king.
brian8871 brian8871 5/20/2021 12:59
Kaito knows which kind of piece, and Toshi knows the color. Toshi says "There is no way either of us could deduce which piece you mean." That eliminates the queen and knight, since they only exist on one side. Kaito says: "Okay, now I know which piece it is!" It couldn't be any of the pawns, since there are multiples on both sides. And if it was a king, Kaito still wouldn't know which color it was. That leaves the bishops. Toshi: "Aha, then I know as well." If it was a white bishop, Toshi wouldn't know which one, which leaves only the black bishop.
Erdmundr Erdmundr 5/19/2021 10:20
A rewording of the implicit logic in the discussion: Toshi: Every piece of my color can also be found in the opposite color. Therefore, neither of us can deduce the answer on our own. Kaito: Toshi's statement implies that they have been told that the color of the piece is White, as I could have been told the piece was a Knight, which can only be found among the Black pieces. Now there is a unique White piece of the type I have been told, so I know what piece the Zen master means. Toshi: Kaito was able to deduce the correct piece by knowing from reading into my statement that it was White. Therefore, there is only one White piece of the type I am looking for. Hence, it is the White King.
TommyCB TommyCB 5/19/2021 08:04
This is similar to a problem I saw as a child around 1968.
In the book "Fun with Puzzles", Joseph Leeming, First Printing 1946, second printing 1968.

The Three Small Boys
Three small boys were talking together when they were joined by an older man. The newcomer noticed that each of the three boys had a smudge of dirt on his forehead.

"Boys," he said, "will each of you look at the foreheads of the other two and if you see a smudge of dirt on either or both, raise your hand." All three boys looked and all three raised their hands.

"Now," said the old gentleman, "if one of you is certain that he has dirt on his own forehead and can tell me how and why he knows this, he is to raise his hand and I will give him a quarter."

The three boys looked at each other for a few moments, and one of them suddenly raised his hand. Can you figure out how he knew that he had a smudge on his forehead?
mdamien mdamien 5/19/2021 07:15
I never considered 1 e8Q+.
ChrisHolmes ChrisHolmes 5/19/2021 06:14
Have you seen this kind of drawing mechanism before? I believe there is an example of this in the book The Joys of Chess, which naturally is by Christian Hesse.
gato90 gato90 5/19/2021 04:30
If Kaito hears Queen or Knight, he would know which piece it is since there are only one of each. Also both are black, so if Toshi hears Black, there would be a chance that Kaito heard Queen or Knight and know the answer. But since Toshi said "There is no way either of us could deduce which piece you mean.", this must mean he heard White instead. Upon hearing this, Kaito realizes Toshi heard White and then he knows the answer. White has only 1 King but two bishops and three pawns, so the only way Kaito can know the answer is if he heard King. Now that Toshi knows that Kaito knows the answer, he as well realizes it must be the White King.
hansj hansj 5/19/2021 03:45
White king, I guess.
1