Retrograde analysis
"Retrograde analysis" sounds daunting, but is a very enjoyable form of recreational chess, requiring humour and lateral thinking. A chess position is considered legal if it can be reached through a regular set of moves. These do not have to be meaningful or strategically sound — just legal. They can be as weird as you like but must lead to the diagram position without breaking any of the rules of chess. And sometimes that is no easy task.
I have always been fascinated by retro problems and have written about them in our puzzle section in the past. I am not sure who invented them, but in the 19th century, the great Samuel Loyd produced a number of problems which needed retrograde analysis. I remember this one fondly:
Samuel Loyd, Musical World, 1859
I was stumped by this problem. I had some good ideas, like 1.Qa1!? and 2.Qh8, but it fails to 1...0-0-0. And there was absolutely no other way to mate in just two moves. At the time I was completely innocent of retrograde analysis and had to look up the solution. It is 1.Qa1! because you can see from the position that the previous black move had to have been with the king or the rook — which means that castling is illegal! So 2.Qh8 does actually mate, and the problem is solved. Beautiful, elegant. And it illustrates a rule we must remember: castling is always permitted in (retro) chess problems unless you can prove that it is illegal.
Two great puzzle composers: Samuel Loyd (1841-1911) and T. R. Dawson (1889-1951)
Thomas Rayner Dawson was an expert in retrograde analysis (and fairy chess). Here is one of his problems I actually solved — and I will leave our readers to do the same:
T. R. Dawson, The Chess Amateur, 1927
Black to move – how did this position arise?
Okay, I'm going to give you a board with the initial position where you can move the pieces and try to achieve the position shown above. Remember in Dawson's position it is Black to move, so the final move must be a white one. Good luck.
 Raymond Smullyan
Raymond Smullyan
The man who brought retroanalytical problems to mainstream attention was Raymond Smullyan (1919-2017), a well-known mathematician, concert pianist, logician, Taoist philosopher, and magician who published a series of books which appeared in Hutchinson in 1980 and later. Siegfried Hornecker wrote about him recently.
Smullyan's books were entitled "Chess Mysteries of..." and the most famous one was of Sherlock Holmes. It was written in the style of the Conan Dolye tales — the problems in this book require the same kind of logic the master detective employed to track down his criminal adversaries. Typically Holmes and Watson would find an interrupted game and discuss how the position on the board came about.
The position on the cover of his first book (click to enlarge) is an introductory puzzle which became one of his most famous compositions. Here's how it is described in the book:
I had a memorable evening alone with Holmes, during which I learned more about retro-analysis than perhaps on any other occasion. "Here, let me set up a little exercise to illustrate the more normal type of situation," he said.
What was Black's last move?
“I call this an ‘exercise', Watson, since it is really too simple to dignify by the word ‘problem'. As you see neither side is mated — nor even in check. The question now is this: Given that Black moved last, what was his last move, and White’s last move?”
I thought for a while, then said, “Holmes, I’m sorry to be such a slow pupil, but the situation again seems impossible! Obviously Black just moved out of check from a7, but I don’t see how White could possibly have moved his bishop to administer the check!”
“Not bad, Watson; not bad at all! I see you are beginning to think. But why do you have this persistent habit of forgetting that a move may involve a capture?”
Then, of course, I saw it. “Right, Holmes, right. Black’s last move was with the king from a7 capturing a white piece on a8. This piece must have moved before that out of the diagonal from g1 to a7 to uncover check from the bishop. What piece could that be? Why obviously a knight, which had moved from b6 to a8. Thus Black’s last move was from a7 to a8, capturing a white knight.”
“Correct,” said Holmes.
A new thought suddenly occurred to me. “Holmes” I said, “is it really necessary in this problem to be given which side was White?”
“Of course,” replied Holmes. “If we hadn’t been given that information, then a second solution would have been possible: A white pawn could have just promoted to bishop.”
 Another interesting book by Smullyan is "Chess Mysteries of the Arabian Knights", which is available on Amazon, for just under $400. Or you can read for free on this web page (PDF). The second would be my choice — but hey, I tend to be a bit frugal. One reader of the book writes: "Mind-bendingly good. Have had endless hours of fun with this." It was not me, but I concur with his opinion completely.
Another interesting book by Smullyan is "Chess Mysteries of the Arabian Knights", which is available on Amazon, for just under $400. Or you can read for free on this web page (PDF). The second would be my choice — but hey, I tend to be a bit frugal. One reader of the book writes: "Mind-bendingly good. Have had endless hours of fun with this." It was not me, but I concur with his opinion completely.
Anyhow you can click on the image on the right to see the cover in a larger size and try to solve the problem, which is also very famous. It is also quite challenging for people who have no experience in retroanalytical problems. Smullyan writes:
It is tempting to give the following fallacious argument that the position is quite impossible regardless of where the White king stands: Either the White king stands on b3 or Black is now in check from the bishop. But the White king can't stand on b3, for if it did, it would be in imaginary (impossible) check from the Black rook and bishop – i.e., it would be simultaneously in check from these two pieces, and whichever just moved to check it, the other one would have been checking it before the move.
Therefore the White king is not now on b3, and Black is in check. How did White give this check? Not by moving the bishop; hence the White king must have moved from b3 to discover the check (it obviously couldn't have moved from c2 ). But if it moved from b3, it would still have been in an imaginary check from the Black rook and bishop. Therefore the position is impossible.
It is amazing how many people will swear by the validity of the above argument! But it is wrong, and for the following reasons. It is indeed true that the White king cannot stand on b3 now, although it does not follow that it could not have just moved from there; it might have just captured a Black piece. How does that help?
I will leave it to the readers to solve this lovely little problem. If you do not succeed (I entreat you to try) then you will find the solution in the game viewer at the bottom of this story.
One more? The following is the second problem from Smullyan's Arabian Knights:
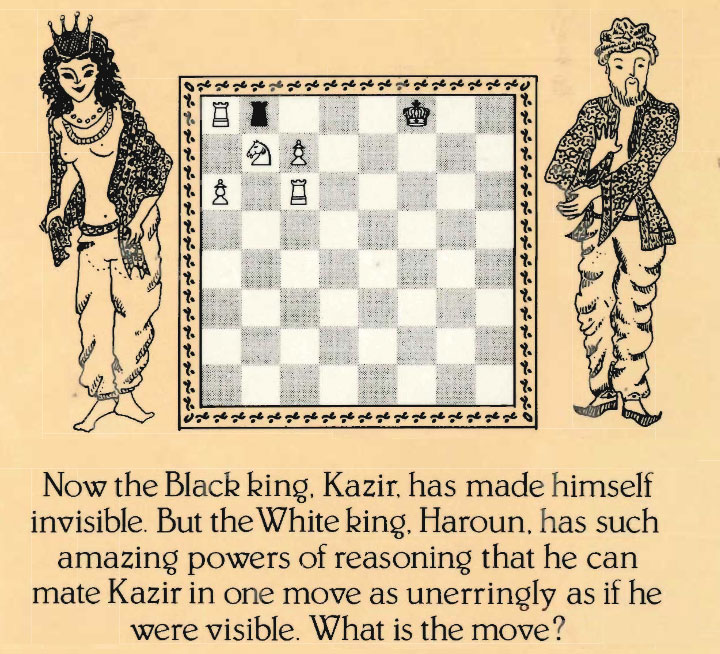
The solution is entitled "Invisible but not Invincible!" There are actually two squares on which the black king can stand. Both allow White to mate in one. You should try to figure it out and only peek at the solution at the bottom of the page if you cannot solve the problem in five minutes.
While we are on invisible pieces here's a clever little problem by Bengt Giöbel, Polis-Tidningen, 1946:
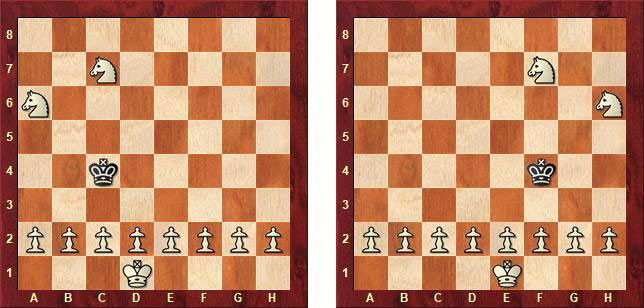
Add a white queen and mate in one, in both positions (solution at the bottom of the page)
Now that you have seen some of the common devices used in retroanalytical problems here are two more for you to solve. The first you will find trivially easy, I'm sure:
Niels Hoëg, Skakbladet, 1916
A very simple position, with just four pieces on the board, and the black king in check. Your task is to reconstruct the last move. It could not have been e5-e6, since the black king would have been in check before the move was played. However, with a little thought, you can, in fact, deduce what the last three moves (two by White and one by Black) must have been. They are unique and must have been played for the above position to have arisen.
Nenad Petrovic, T.T. Problem 1953-54
What were the last three moves?
In this rather odd position, you are asked for the last moves that were played. To make it clear: what were the last three moves by White and the last three moves by Black (= six half-moves)? Naturally, everything has to be legal.
The above problems are meant as an introduction for readers who have very little experience with the subject. There are many more complex positions, to which we will come at a later date. To check if you have got the hang of it here is a (fairly simple) problem for you to solve:
Werner Keym, Die Schwalbe, 1979
In the above position, it is clearly Black to move, because his king is in check. What you have to figure out is how the position could arise, with only legal chess moves. It is obvious, you will say, that White has just played his knight from b4 to a2 giving check, but then what could Black's move before that have been? Find a clearly legal position which can give rise to the diagram in two or three moves. I worked it out myself and had a lot of fun doing so.
Okay, one last one (while I'm on a roll)?
N.G.G. van Dijk, Østlenddingen 1959
The solution is quite obvious — in fact, there are two possible first moves: 1.0-0 and 1.Kf2. But try to enter them in our active diagram and you will see that only one is legal, i.e. you will not be able to enter the other. Why?
Solutions
| Replay and check the LiveBook here |
Please, wait...
1.Nf3 Nf6 2.Nh4 Ne4 3.Nf3 Ng5 4.Nh4 Nh3 5.Rg1 Nxg1 6.Nf3 Nh3 7.Nh4 Nf4 8.Nf3 Nh5 9.Nh4 Nf6 10.Nf3 Ng8 11.Ng1 - Start an analysis engine:
- Try maximizing the board:
- Use the four cursor keys to replay the game. Make moves to analyse yourself.
- Press Ctrl-B to rotate the board.
- Drag the split bars between window panes.
- Download&Clip PGN/GIF/FEN/QR Codes. Share the game.
- Games viewed here will automatically be stored in your cloud clipboard (if you are logged in). Use the cloud clipboard also in ChessBase.
- Create an account to access the games cloud.
| Dawson | - | Retro problem | - | | 1927 | A05 | The Chess Amateur | |
| Smullyan,R | - | Retro problem | - | | 1980 | | Chess Mystries of Sherlock Holmes | |
| Smullyan,R | - | Retro problem: where's the bK? | - | | 1981 | | Chess Mysteries of the Arabian Knights | |
| Hoëg,N | - | Retro problem | - | | 1916 | | Skakbladet | |
| Petrovic,N | - | Retro problem | - | | 1953 | | T.T.Problem | |
| Keym,W | - | Retro: Last move? | - | | 1979 | | Die Schwalbe | |
| van Dijk,N | - | Mate in two (retro problem) | - | | 1959 | | Østlenddingen | |
Please, wait...
Solution to the Giöbel problem: in both positions, all white pawns are on the board and hence there can be no promoted white queen involved. The invisible queen could not have left the first rank, and could not have crossed over the king to the kingside. So in the first position the queen could only legally be on a1, b1 or c1. Only from a1 is a mate in one move possible: 1.b3#. In the mirror position the same logic applies, with the white queen on a1, b1, c1 or d1. She must be on c1 to allow 1.d3#.




















 Another interesting book by Smullyan is "Chess Mysteries of the Arabian Knights", which is available on
Another interesting book by Smullyan is "Chess Mysteries of the Arabian Knights", which is available on 







