Longest dual-free direct mate problem

Where is the white king?

In our weekend puzzle, which Ken Thompson thought up in 1986, when he was fooling around with his first five-piece tablebase endings, we asked you to find the only position on which the white king can be placed in the above position so that White (to play) can force a win. There is really only one square that allows this – put the white king on any other and Black is able to force a draw. Here is the solution:

Mate in 203 moves

By John Nunn

Long-range mating problems are not generally the strong point of computers, especially if the number of moves they are required to find are counted in dozens or over a hundred. But in spite of this it is possible to use a computer program when solving such problems. They usually work with shorter threats that computers can understand, and such threats can easily be found with a chess engine. The overall long-range strategy may escape them completely, but it is here that the human operator can jump in.

The longest dual-free direct mate problem

The longest direct mate problem was devised by the Danish composer Walther Jörgensen, who published it in the problem magazine "Schwalbe" in 1976 as a mate in 200 moves. In the same year André Chéron found a small change that extended the problem to 203 moves. Subsequently there were attempts to extend Jörgensen's problem to 210 or even 215 moves, but this came with a weakening of the black forces, so that it was possible that White could win the position by conventional play in a shorter number of moves.

Longer direct mate problems have been published, like a mate in 257 by O. T. Blathy, and one by the same author in 290 moves. But these problems all contained duals, which means that at certain points in the solution the white continuation is not forced. In particular, many of these very long problems involve repeated king marches by White in order to lose a tempo. These marches have a triangulation in them at some point (for example, Kb1-a1-a2-b1), so that it will be Black to move at the end of the march. Generally, these triangulations involve a dual in that they can be executed in two ways, for example Kb1-a1-a2-b1 or Kb1-a2-a1-b1. The beauty of the Jorgensen problem is that there are no such alternatives.

This is precisely why the Jörgensen problem is so remarkable: every single one of the 203 moves is forced (assuming optimum defence by Black), and each white move needs to be executed in exactly the sequence given. It is in my opinion the longest dual-free direct mate problem without pawn promotions.

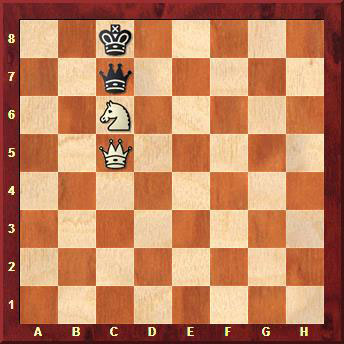
Walter Jörgensen, Die Schwalbe 1976

White to play and mate in 203 moves

We immediately see that due to the material superiority of the opponent White cannot win by conventional means. He must execute a forced mating attack. The first task, however, is to blockade the black pawn on h3 and gain total control of the position. So let us begin [a full Javascript replay is provided at the end of this article]:

1.Qg1+ Kf3 2.Qf1+ Kg4 (2... Ke4 3.Qd3 mate) 3.Qe2+ Rf3 4.Qe6+ Rf5 5.Bh2.

With the introductory moves White has tied down the position so that the opponent's forces can no longer be mobilized. We take note that Black cannot move either of his knights: if he moves the Ng6 then White mates immediately with Ne5; the move 5...N8e7 allows 6.Qe2+ Rf3 7.Qe4+ Rf4 8.Nf6#; 5...Nf6? just delay the end by one move: 6.Nxf6+ Kf3 7.Qe3+ Kg2 8.Qg1+ Kf3 9.Qf1#.

There is only one continuation for Black: 5...Kf3. This threatens to release the black heavy pieces, which White must avoid at all costs. 6.Qe3+ Kg2 7.Qg1+ Kf3 8.Qf1+ Kg4 9.Qe2+ Rf3 10.Qe6+ Rf5. We will call these five moves the "Jörgensen Manoeuvre". They have led to the position after White's fifth move (see diagram above), but with White to move.

What can White do with the free move? If Black had no pawns on the a and c-files the game would soon be over: White executes the Jörgensen Manoeuvre three times, bringing his king to d1 in the process, after which Black cannot play ...Kf3 any more, because Qe2 would be mate. He has to move a knight which leads to mate as described above.

You may think that White could simply go after the black pawns after each Jörgensen Manoeuvre, but note that moving the king to a4 leaves it open to a rook check when the black king moves to f3; and playing c3-c4 will make the white king vulnerable to rook checks on the third rank, which it could thus no longer occupy. No, the process is much longer and more convoluted.

11.Kb2 Kf3 12.Qe3+ Kg2 13.Qg1+ Kf3 14.Qf1+ Kg4 15.Qe2+ Rf3 16.Qe6+ Rf5 17.Kc1 Kf3 18.Qe3+ Kg2 19.Qg1+ Kf3 20.Qf1+ Kg4 21.Qe2+ Rf3 22.Qe6+ Rf5 23.Kd1

Now Black must move a pawn (23...Kf3? 24.Qe2#) 23...a4. This offers the greatest resistance. 24.Kc1 24.Ke1? allows the a-pawn to promote. 24...Kf3 25.Qe3+ Kg2 26.Qg1+ Kf3 27.Qf1+ Kg4 28.Qe2+ Rf3 29.Qe6+ Rf5 30.Kd1 a5. After 30...a3? 31.Kc1, 37.Kb1, 43.Ka2, 49.Kxa3, 55.Kb2, 61.Kc1 White can make three king moves without the Jörgensen Manoeuver (67.Kd1 a5 68.Ke1! a4 69.Kd1) saving a total of ten moves.

31.Kc1 Kf3 32.Qe3+ Kg2 33.Qg1+ Kf3 34.Qf1+ Kg4 35.Qe2+ Rf3 36.Qe6+ Rf5 37.Kd1 a6 38.Kc1 Kf3 39.Qe3+ Kg2 40.Qg1+ Kf3 41.Qf1+ Kg4 42.Qe2+ Rf3 43.Qe6+ Rf5 44.Kd1 a3.

The first "lemming suicide plunge": the black pawn has had to move to a square on which it can be safely captured – after four Jörgensen Manoeuvres: 45.Kc1 ... 51.Kb1 ... 57.Ka2 ... 63.Kxa3.

Now the white king must return to d1 to force another pawn move: 69.Kb2 ... 75.Kc1 ... 81.Kd1 a4. Now White uses the Jörgensen Manoeuvre to shuffle his king between c1 and d1, forcing black pawn moves: 82.Kc1 ... 88.Kd1 a5 89.Kc1 ... 95.Kd1 c4. Note that after the next Jörgensen, 96.Kc1 Kf3 97.Qe3+ Kg2 98.Qg1+ Kf3 99.Qf1+ ...

... Black can try 99...Ke4, since White can no longer mate with Qd3. But 100.d3+ does the job: 100...Kd5 (100...Ke3 101.Bg1+ Rf2 102.Qxf2#) 101.Qxf5+ Ne5 102.Qxe5#.

So: 99...Kg4 100.Qe2+ Rf3 101.Qe6+ Rf5 102.Kd1 c5 103.Kc1 Kf3 104.Qe3+ Kg2 105.Qg1+ Kf3 106.Qf1+ Kg4 [106...Ke4? Black can try to use the free c6 square by 106...Ke4?, but this proves in vain after 107.d3+ Kd5 108.Qxf5+ Kc6 109.Qe6+ Kb7 110.Qb6+ Kc8 111.Qc7#.]. 107.Qe2+ Rf3 108.Qe6+ Rf5

White still cannot pick up the pawn on a4: 109.Kb2? Kf3 110.Qe3+ Kg2 111.Qg1+ Kf3 112.Qf1+ Kg4 113.Qe2+ Rf3 114.Qe6+ Rf5 115.Ka3 Kf3 116.Qe3+ Kg2 117.Qg1+ Kf3 118.Qf1+ Kg4 119.Qe2+ Rf3 120.Qe6+ Rf5 121.Kxa4 Kf3 122.Qe3+ Kg2 123.Qg1+ Kf3 124.Qf1+ Ke4! 125.Qe1= (125.Qxc4+ Kf3-+ The queen is pinned!; 125.d3 Ke3–+).

109.Kd1 a3 110.Kc1 ... 116.Kb1 Kf3 117.Qe3+ Kg2 118.Qg1+ Kf3 119.Qf1+ Ke4!

This forces White to capture the pawn on c4, giving the pawn c5 an extra move. 120.Qxc4+ [120.d3+? Ke3!–+] 120...Kf3 121.Qf1+ Kg4 122.Qe2+ Rf3 123.Qe6+ Rf5 124.Ka2 ... 130.Kxa3 ... 136.Kb2 ... 142.Kc1 ... 148.Kd1 a4 149.Kc1 ... 155.Kd1 c4 156.Kc1 ... 162.Kd1 a3 163.Kc1 ... 169.Kb1 ... 175.Ka2 ... 181.Kxa3 ... 187.Kb2 Kf3 188.Qe3+ Kg2 189.Qg1+ Kf3 190.Qf1+ Ke4. Black extends the solution by two moves. 191.Qxc4+ Kf3 192.Qf1+ Kg4 193.Qe2+ Rf3 194.Qe6+ Rf5 195.Kc1 Kf3 196.Qe3+. The Jörgensen Manoeuvre is executed for the last time. 196...Kg2 197.Qg1+ Kf3 198.Qf1+ Kg4 199.Qe2+ Rf3 200.Qe6+ Rf5 201.Kd1

The final zugzwang. 201...N8e7 [201...Kf3 202.Qe2#] 202.Nf6+ Kf3 203.Qe2#.

Solving in Style

The above article was written by John Nunn for a German computer chess magazine I was editing at the time. It was not extracted from one of my all-time favourite chess books, though it would have been well suited to be part of it.

The book was given to me in late 1986 by the author, as you can see from the dedication above. On the left is the original hardcover by George Allen & Unwin, on the right the 2002 reprint by Gambit, which I am pleased to see is still available for purchase, for £9.50/$14.95/€12.85.

In this book, John Nunn (who recently turned 60), a top-class grandmaster and also one of the world's finest solvers, explains the methods by which chess problems and studies can be tackled. The logical and creative methods advocated, while targeted at the solving of composed positions, may also help players find startling tactical solutions in their games. Solving in Style also constitutes an entertaining and insightful introduction to the world of problems and studies. "Nunn does an excellent job of explaining the special rules of this parallel universe, but where he is likely to gain the most converts is in the field of chess studies," wrote John Donaldson. "Many of these studies look sufficiently 'game-like' that the over-the-board player will have no trouble appreciating the beauty and ingenuity involved. Highly recommended."

I cannot tell you how many hours I have spent with this book, which Jon Speelman with a penchant for Cockney rhyming slang referred to as "Dissolving in Bile". At the time I would use a chess board, soon thereafter transferring the positions into chess computers and problem solving programs. I do hope that someday John will make the problems in the book available in PGN – how many hours we would spend playing through the solutions on the GUI of a modern chess program.

Frederic Friedel