Winning starts with what you know
The new version 18 offers completely new possibilities for chess training and analysis: playing style analysis, search for strategic themes, access to 6 billion Lichess games, player preparation by matching Lichess games, download Chess.com games with built-in API, built-in cloud engine and much more.
Recently, it occurred to me that some chess problems might be more interesting if the stipulation (e.g., ‘White to Play and Mate in 3’) also held true for the opponent. This would mean that in a position where White was to play and mate in n moves, yet if Black was to play instead from the same position, it would also be mate in exactly n moves. A chess problem that could satisfy both stipulations, i.e., ‘White (or Black) to Play and Mate in N’, would tend to be more difficult to compose and challenging to solve. A ‘two in one’ problem, if you will. We may call these, ‘mutual mate’ problems or, in the case of a study, a ‘mutual win’.
I could not find anything in the existing chess problem literature which already described them. ‘Duplex’ is perhaps an umbrella term that covers them but, to my knowledge, it is not specific to direct mates of the same length for both sides and seems to apply largely to helpmates.
Therefore, I decided to perform an experiment just to see how common such things were ‘in the wild’, so to speak. In fairness, I am confident that if human composers actually tried to compose aesthetically pleasing and thematic direct mate problems of this type, they would succeed as well. Not being much of a composer myself, I turned to my computationally creative computer program, Chesthetica, and its 3,473 compositions from July 2010 to the present (consisting of mates in 2, 3, 4, 5 and studies) which I had been selecting for and compiling. Interested readers can find more information about Chesthetica (pictured below) at the official website.
These 3,473 autonomously computer-generated compositions are the ones I happen to have examined and found aesthetically pleasing, interesting or educational over the years. There are actually presently over 110,000 compositions by Chesthetica; most of which I have never even seen due to the sheer volume. Many more were simply lost as I had discarded the ones I did not see or like for some years prior as well. Only later did it occur to me that it might be prudent to keep them all regardless. The smaller composition set was nonetheless a reasonable sample to test for the new type of chess problem I am describing in this article. While Chesthetica was never explicitly programmed to compose such problems, they might still have occurred. I programmed a subroutine into Chesthetica which would detect mutual mates; however, studies were excluded for now given that mates are decisive and would better illustrate the concept.
From a total of 3,473 compositions, 294 were studies so the remainder of forced mates (in 2, 3, 4 and 5 moves) was 3,179 or about 91.5%; still a sufficiently large sample, in my estimation. The number of mutual mates detected was only 29 or less than 1%. It is difficult to tell if this is low, average or high, but I suspected the frequency of mutual mates would likely be even lower in a sample taken from traditional (i.e., typically human composed) direct mate chess problems or a sample of forced mate endings from tournament games. A random sample of 3,179 direct mate problems by human composers (from a collection of 29,453 forced mates in 3, which I happened to have) revealed only two mutual mates or less than 0.1%. Keep in mind that neither Chesthetica nor the human composers ‘intentionally’ composed these as mutual mates but instead with the stipulation that White is to play and force mate in n moves. It just so happens that in these 29 compositions, and two human compositions, if Black was to play instead, it would also be a mate in the same number of moves.
Also note that Chesthetica does not compose traditional chess problems but rather chess constructs (a type or class of chess problem) which means they do not necessarily have to conform to traditional chess composition conventions, e.g., not having a check in the first move. Put simply, chess constructs cover a larger spectrum of compositions and can lie anywhere between traditional chess problems and sequences typically found in real games. This casts the widest net in terms of aesthetics. Besides, if there’s anything I’ve learned about human aesthetic perception in chess over the years, at least primarily via the many chess communities on Facebook, is that people tend not to ‘like’ compositions they find too difficult to solve.
In any case, the positions below show four examples of ‘mutual mates’ I chose from the 29 detected, and the two detected from human compositions. Incidentally, readers are also free to judge for themselves how aesthetically pleasing and thematic these are in contrast to the computer-generated ones. Try to solve for White first and then Black in the same number of moves, as per the modified stipulation. The solutions are provided at the end of the article.
White (or Black) to Play and Mate in 5 |
White (or Black) to Play and Mate in 5 |
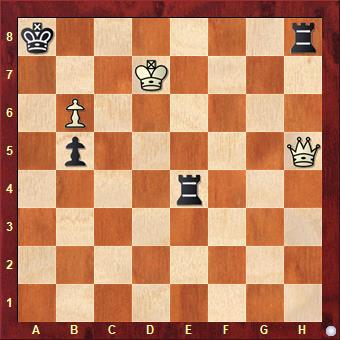
White (or Black) to Play and Mate in 3 |
White (or Black) to Play and Mate in 3 |
Selection of Incidental ‘Mutual Mate’ compositions by Chesthetica
|
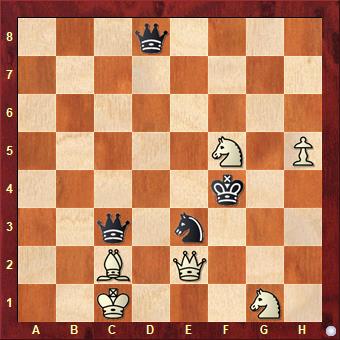
White (or Black) to Play and Mate in 3 |
White (or Black) to Play and Mate in 3, |
‘Mutual Mate’ compositions by human composers
The concept of a mutual mate or win should, I hope, be clear at this point. While the examples shown above may not conform to many traditional composition conventions or lack aesthetic appeal to some, this type of chess problem does not preclude any further conditions one may wish to apply. For instance, it could be specified to the composer that both the White to play and Black to play solutions should demonstrate the same theme and that neither should have any (major) duals either. It may also be specified that both the White and Black armies have the same amount or type of material. At some point, I would imagine a particular type of mutual mate may even rival the difficulty of creating a Babson task.
Mutual ‘wins’ (pertaining to studies), naturally, would generally be harder to compose compared to the direct mate variety, especially given that the wins should be decisive or clear at approximately, if not exactly, the same length. I have not programmed Chesthetica to explicitly aim for any of these, but if the idea catches on, I might at some point. Perhaps the reader, experienced composer or otherwise, would now like to try composing a mutual mate of their own.
Here are the solutions to the above examples:
We would like our readers to try their hand at composing Mutual Mate positions. Please submit any positions you might come up with to the editors.
