December 28, 2015: The Pervakov study
By Frederic Friedel
Yesterday we told you how Garry Kasparov had given ten-year-old chess prodigy Vincent Keymer a study to solve, one that looked deceptively simple. After that the lad got a very subtle and instructive endgame lesson from the 13th World Champion, in form of a study which we asked you to solve.
Olge Pervakov, 4.p Moscow Sports Committee Ty Bulletin 1986
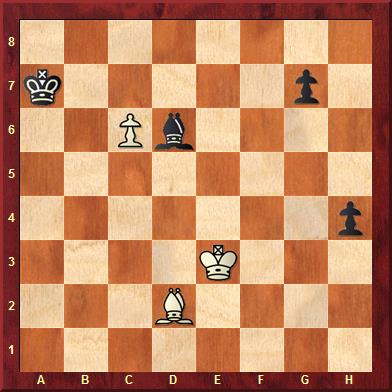
White to play and draw

After forcing Vincent to work on it in his head, in the end in fact without the pieces,
Garry sat down with the boy to analyse the study, and to explain important endgame concepts.

These are really lessons of the highest possible calibre, deeply inspiring to the chess prodigy
Vincent understood the study, and when we asked him to annotate it for us he did so without any problems. Our intention was to give you his analysis as the solution, but there was one problem: his notes were a bit difficult for normal amateurs to follow. The ten-year-old assumes that anyone can understand his evaluations, like "...leading to a draw" (but how, Vincent??). So we asked an experienced chess teacher, ChessBase editor Sagar Shah, to add some explanatory notes, for less experienced students and amateurs. If you now go through the following solution carefully you will learn quite a bit about chess endings and tempos. Don't miss it – there is no harm in adding some Elo points to your rating!
| Replay and check the LiveBook here |
Please, wait...
1.Kf2! 1.Kf3? h3 2.Bh6 gxh6! 3.Kf2 Bh2! 4.Kf3 h5! 1...h3! 1...Kb6 2.Kg2 Kxc6 3.Kh3 Be7 4.Be1 g5 5.Bd2 Kd5 6.Bxg5= 2.Bh6‼ 2.Be3+ Ka6 3.c7 Bxc7 4.Bh6 4.Kf3 Kb5 5.Bg1 h2 6.Bxh2 Bxh2 7.Kg4 Be5 4...g6 5.Be3 Kb5 6.Kf3 Kc4 7.Bg1 Kd5 2...g6 2...gxh6 3.Kg1= 3.Be3+! 3.Kf3 Kb6 4.Be3+ Kxc6 5.Bg1 Kd5 6.Kg4 Ke4 7.Kxh3 Kf3! 3...Ka6 4.c7 4.Kf3 Kb5 5.Bg1! Kxc6 5...h2 6.Bxh2 Bxh2 7.Kg4= 6.Kg4 Bc5! 6...Be5 7.Kxh3= 7.Bh2!= 7.Kxh3? Bxg1 8.Kg4 Be3+- 4...Bxc7 5.Kf3 Kb5 6.Bg1 h2 6...Kc4 7.Kg4 Bd8 8.Kxh3 7.Bxh2 Bxh2 8.Kg4! ½–½
- Start an analysis engine:
- Try maximizing the board:
- Use the four cursor keys to replay the game. Make moves to analyse yourself.
- Press Ctrl-B to rotate the board.
- Drag the split bars between window panes.
- Download&Clip PGN/GIF/FEN/QR Codes. Share the game.
- Games viewed here will automatically be stored in your cloud clipboard (if you are logged in). Use the cloud clipboard also in ChessBase.
- Create an account to access the games cloud.
| Pervakov,O | - | White to play and draw | - | ½–½ | 1986 | | 4.p Moscow Sports Committee Ty Bulletin | |
Please, wait...
Now for the final problem Vincent was given during that memorable two-hour session in Berlin:

It was a delightful puzzle which everyone present in Garry's suite thoroughly enjoyed
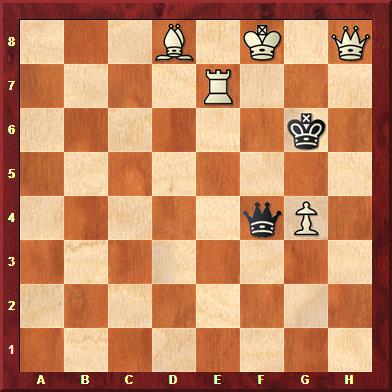
White to play and win
Yes, White is in check, and he has just four legal moves (one of which, 1.Rf7, lead to an immediate mate with 1...Qxf7#. So which of the other three should White play? Try to work this out in your head, and remember: it is quite appropriate to grin in delight, as Vincent did, when you find the winning line.
Pal Benko's twins
The first mate-in-two problem published on the 26th was the following:
Pal Benko, 2015
White to play and mate in two.
The next twin involves moving the knight g1 to e3:
Pal Benko, 2015

Mate in two moves
Once again we ask our readers to solve the problem and then construct a new position by moving or inserting a single piece on a different square. The new problem must again be a mate in two, with a different solution. And it must have no "cooks" – multiple solutions that will spoil an chess problem.






























